Beyond nodes and edges
Since Euler’s solution on the Seven Bridges problem, mathematical graph theory has become a useful tool in various fields, including neuroscience.
Makliya Mamat / April 28, 2024
Graph Theory, one of the branches of mathematics, is undeniably one of the most powerful tools of applied mathematics with remarkable interdisciplinary applicability in diverse areas, spanning from social and political sciences, to biology, chemistry, neuroscience and astrophysics and cosmology and stands out as a foundational concept of Network Science.
In our recent study titled ‘Beyond Nodes and Edges: A Bibliometric Analysis on Graph Theory and Neuroimaging Modalities,’ published in Frontiers in Neuroscience, we discussed its interdisciplinary significance by exploring its intersection with neuroimaging modalities.
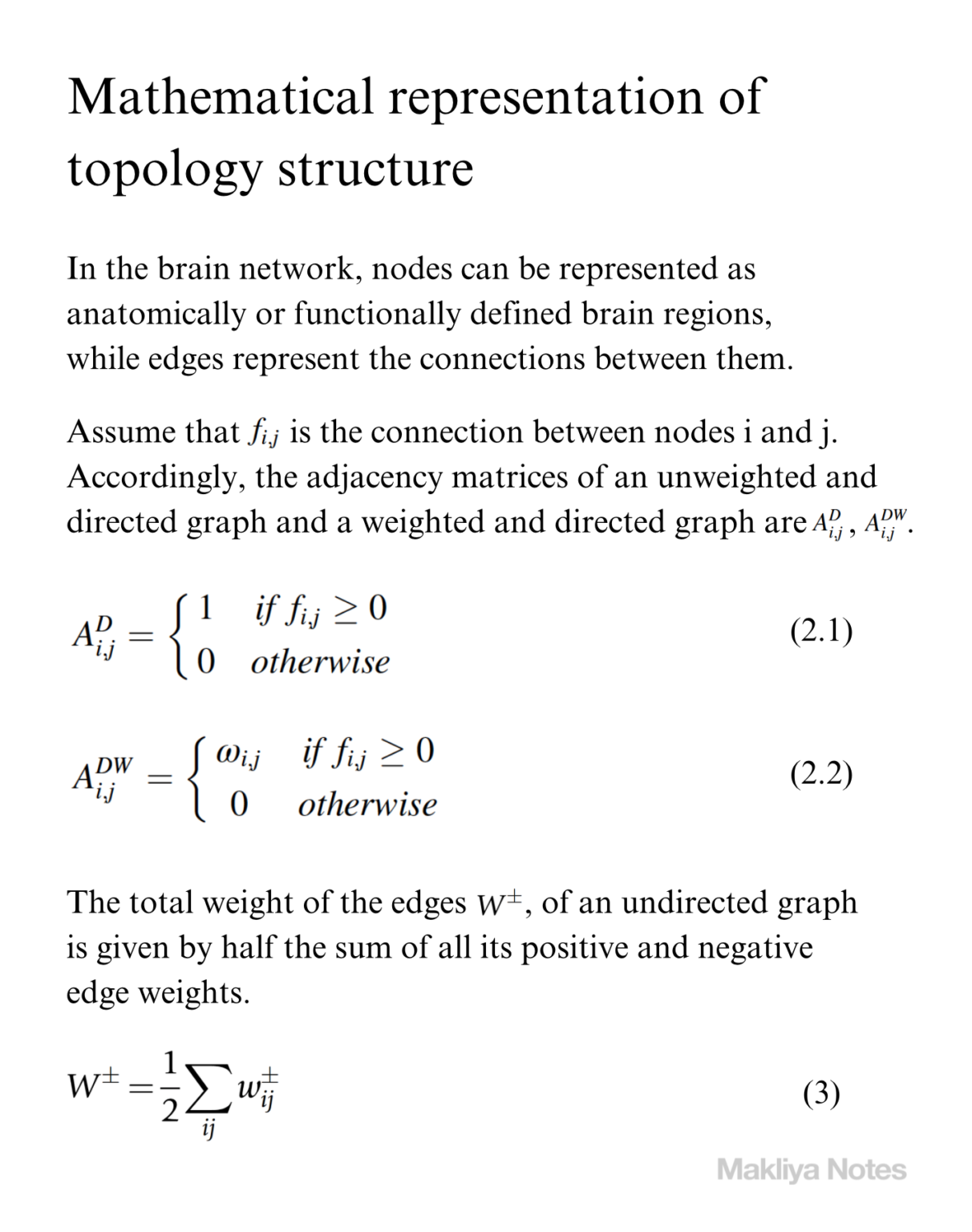
Graphs are mathematical structures that represent any type of entities which can be related to each other via pairwise relations. Each entity is represented by node (vertex) of the graph. Pairwise relations between entities are represented via edges (links) connecting pairs of corresponding nodes.
Additionally, a numerical value (weight) can be associated with each edge, which acts as an indicator of the strength of the relation between the corresponding nodes. For similarity graphs in particular, weights quantify the degree of similarity between the endpoints of their edges.
The human brain has long been a subject of fascination and exploration. Understanding the brain’s connectivity patterns and dynamic interplay between its components holds the key to comprehending cognition, behavior, and various neurological disorders.
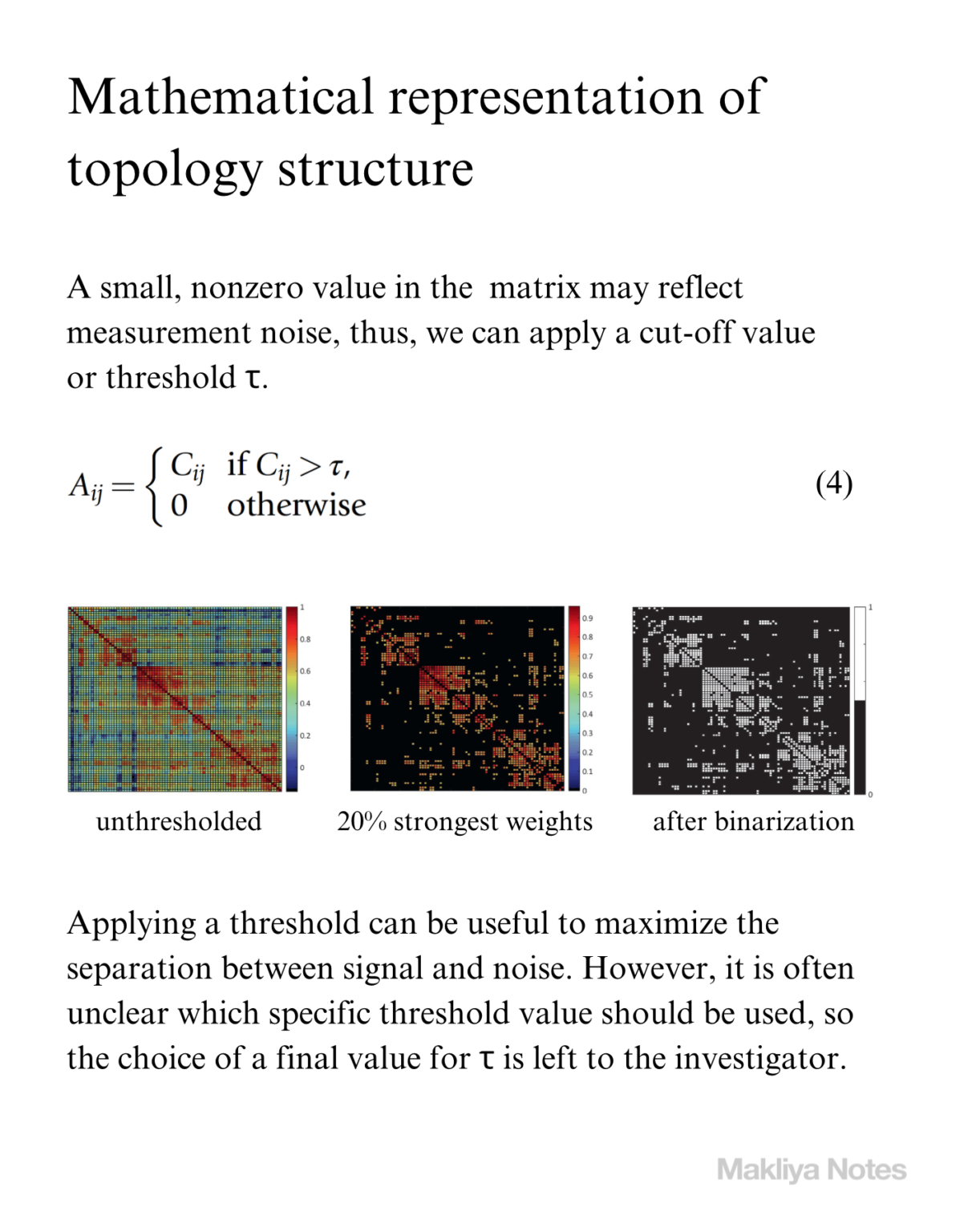
The convergence of graph theory and neuroimaging has ushered in a transformative paradigm in brain research. By applying graph theoretical approaches to neuroimaging data, researchers can uncover fundamental principles governing brain network organization, such as the presence of highly connected “hub” regions and the modular organization of functional brain networks.
Our study showed the evolving landscape of brain network research. Through comprehensive data curation and visualization tools, we mapped out the trajectory and trends within this multidimensional domain.
The expanding frontier of statistical mechanics in complex networks, alongside with the robust tools of graph theory analysis, play an important role in deepening our understanding of the brain’s connectome. By identifying gaps and prospective pathways, we hope to provide insights for future advancements in this field.

Contents
Newsletter
- Updates from Makliya Notes will be delivered to your inbox.